How To Write A Good Laboratory Report
Table of Contents
How To Write A Good Laboratory Report

SAMPLE LAB INSTRUCTION
EXPERIMENTAL INVESTIGATION OF C/D
Introduction: How is the circumference of a circle related to its diameter? In this lab, you design an experiment to test a hypothesis about the geometry of circles. This activity is an introduction to physics laboratory investigations. It is designed to give practice taking measurements, analyzing data, and drawing inferences without requiring any special knowledge about physics.
EQUIPMENT (PER GROUP):
- Metric ruler
- Vernier calipers
- At least 5 objects with diameters ~1 cm to ~10 cm: (penny, marble, “D” cell, PVC cylinders)
PROCEDURE:
Design an experimental procedure to test the following hypothesis:
Hypothesis: The circumference (C) of a circle is directly proportional to its diameter (D).
Make sure you record what you do as you do it, so that the procedure section of your report accurately and completely reflects what you did. Some helpful hints for taking and recording data are in the lab tips and in the grading rubric.
ANALYSIS:
Note: As the semester progresses, you will be expected to take more and more responsibility for deciding how to analyze your data. Drawing valid inferences from data is a vital skill for engineers and scientists. The instructions for analyzing data for most labs will not be as detailed as the instructions below.
- Numerical Analysis: Calculate the ratio C/D for each object. Estimate the precision of each value of C/D.
- Graphical Analysis: Use Excel to construct a graph of C versus D. Use Excel to display the equation of the best fit line through your data. Use the LINEST function to estimate the uncertainty in the slope and intercept of the best fit line. Make sure you interpret the meaning of both the slope and intercept. A checklist for graphs is in the grading rubric.
- Questions to consider:
- How do your calculations and graph support or refute the hypothesis?
- Does your graphical analysis agree with your calculations?
- Do your results for the C/D ratio agree with accepted theory?
REPORT:
A sample lab report for this activity is provided as an example for you to follow when writing future lab reports.
SAMPLE LAB REPORT: EXPERIMENTAL INVESTIGATION OF C/D
ABSTRACT
In this investigation, we examined the hypothesis that the circumference (C) and diameter (D) of a circle are directly proportional. We measured the circumference and diameter of five circular objects ranging from 2 cm to 7 cm in diameter. Vernier calipers were used to measure the diameter of each object, and a piece of paper was wrapped around each cylinder to deterimine its circumference. Numerical analysis of these circular objects yielded the unitless C/D ratio of 3.14 ± 0.03, which is essentially constant and equal to pi. Graphical analysis lead to a less precise but equivalent estimate of 3.15 ± 0.11 for this same ratio. These results support commonly accepted geometrical theory which states that C = p D for all circles. However, only a narrow range of circle sizes were analyzed, so additional data should be taken to investigate whether the constant ratio hypothesis applies to very large and very small circles.
INTRODUCTION
PROCEDURE:
Five objects were chosen such that measurements of their circumference and diameter could be obtained easily and would be reproducible. Therefore, we did not use irregularly shaped objects or ones that could be deformed when measured. The diameter of each of the 5 objects was measured with either the ruler or caliper. The circumference and diameter of each object was measured with the same measuring device in case the two instruments were not calibrated the same. The circumference measurement was obtained by tightly wrapping a small piece of paper around the object, marking the circumference on the paper with a pencil, and measuring this distance with the ruler or caliper. The uncertainty specified with each measurement is based on the precision of the measuring device and the experimenter’s estimated ability to make a reliable measurement.
EQUIPMENT USED:
- “D” cell battery, 2 short pieces of PVC pipe, tomato soup can, penny coin
- Metric ruler with millimeter resolution
- Vernier caliper with 0.05 mm resolution
| Object Description | Diameter (cm) |
Circumfer. (cm) |
Measuring Device |
| Penny coin | 1.90 ± 0.01 | 5.93 ± 0.03 | Vernier caliper, paper |
| “D” cell battery | 3.30 ± 0.02 | 10.45 ± 0.05 | Vernier caliper, paper |
| PVC cylinder A | 4.23 ± 0.02 | 13.30 ± 0.03 | Vernier caliper, paper |
| PVC cylinder B | 6.04 ± 0.02 | 18.45 ± 0.05 | Plastic ruler, paper |
| Tomato soup can | 6.6 ± 0.1 | 21.2 ± 0.1 | Plastic ruler, paper |
ANALYSIS:
The C/D value for the penny is (5.93 cm)/(1.90 cm) = 3.12 (no units). The precision of the ratio can be estimated using the error propogation formula:
Results for all five objects are given in the table below.
| Object Description | Diameter (cm) |
Circumfer. (cm) |
C/D calculated (no units) |
| Penny | 1.90 ± 0.01 | 5.93 ± 0.03 | 3.12 ± 0.02 |
| “D” cell battery | 3.30 ± 0.02 | 10.45± 0.05 | 3.17 ± 0.02 |
| PVC cylinder A | 4.23 ± 0.02 | 13.30 ± 0.03 | 3.14 ± 0.02 |
| PVC cylinder B | 6.04 ± 0.02 | 18.45 ± 0.05 | 3.06 ± 0.01 |
| Tomato soup can | 6.6 ± 0.1 | 21.2 ± 0.1 | 3.21 ± 0.05 |
Average C/D = 3.14 ± 0.03, where 0.03 is the standard error of the 5 values.
From this empirical investigation, the average C/D ratio is 3.14 ± 0.03 (no units). This ratio agrees with the accepted value of pi (3.1415926…). The uncertainty associated with the average C/D ratio is the standard error of the five C/D values, which is equal to the standard deviation (0.06) divided by the square root of N, which in this case is 5 since there were five measurements.
While the five C/D values do not agree within their estimated uncertainties, the variation between these values is relatively small (only about 0.06/3.14 = 2%), which suggests that the C/D ratio is a constant value. The reason for the imperfect agreement may be that the individual uncertainties were underestimated or perhaps is a consequence of the “paper” method used for measuring the diameters of the object. The paper may have slipped while we made the mark, but this “slip effect” should only be a random error, which would not affect the average value of our measurements for C, since there is no reason to believe that the paper would have consistently slipped in the same direction (either too high or too low) every time.
Another way to visualize and calculate this constant circle ratio is by graphing the circumference versus diameter for each object. Graphs are especially useful for examining possible trends over the range of measurements.
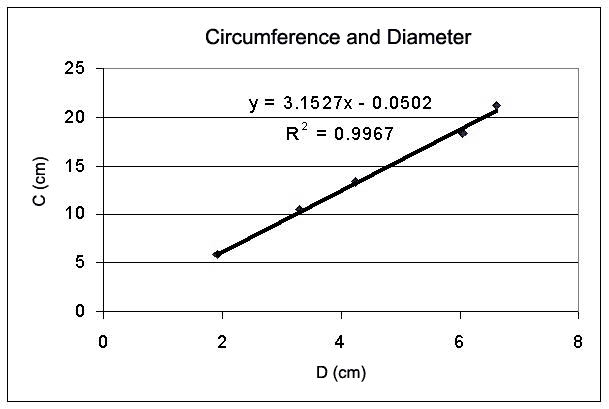
If C is proportional to D, we should get a straight line through the origin. From our numerical results, we would expect the slope of the C vs. D graph to be equal to pi. The slope of the best fit line is (3.15 ± 0.11), which is equal to pi within its uncertainty. The intercept is essentially zero: (-0.05 ± 0.5). The R squared statistic shows that the data all fall very close to the best fit line. If all the data lie exactly on the fitted line, R squared is equal to 1. If the data are randomly scattered, R squared is zero. With an R^2 value of 0.997, our linear equation appears to fit the data very well.
DISCUSSION
Our results support the original hypothesis for 5 circles ranging in size from 2 cm to 7 cm in diameter. The C/D ratio for our objects is essentially constant (3.14 ± 0.03) and equal to pi. The specified uncertainty is the standard error of the C/D ratio for the five objects. Graphical analysis also supports the “directly proportional” hypothesis. The line has an intercept (-0.05 ± 0.5) that is equal to zero within the uncertainty and a slope (3.15 ± 0.11) equal to pi . The larger uncertainty from the graphical analysis suggests that the random measurement errors may be larger than estimated in the numerical analysis. A more extensive investigation of this C/D relationship over a wider range of circle sizes should be performed to verify that this ratio is indeed constant for all circles.
The uncertainty in the measurements could be due to the paper-wrapping method of measuring the circumference, circles that may not be perfect, and the limited precision of the measuring devices. The use of paper to measure the circumference was probably the most significant source of uncertainty. It is unlikely, however, that this measurement technique biased our results, since the technique probably gave measurements of C that were too high in some cases and too low in others.
The C/D ratio for a perfect circle was defined long ago by the Greek symbol: pi = 3.14159… Our measured value appears to be consistent with the accepted value of pi within the limits of our experimental uncertainty. This unique C/D ratio has many important applications wherever circles or spheres are encountered.
Copyright Warning: Contents on this website may not be republished, reproduced, redistributed either in whole or in part without due permission or acknowledgement. All contents are protected by DMCA.
The content on this site is posted with good intentions. If you own this content & believe your copyright was violated or infringed, make sure you contact us via This Means to file a complaint & actions will be taken immediately.